Net Present Value (NPV)

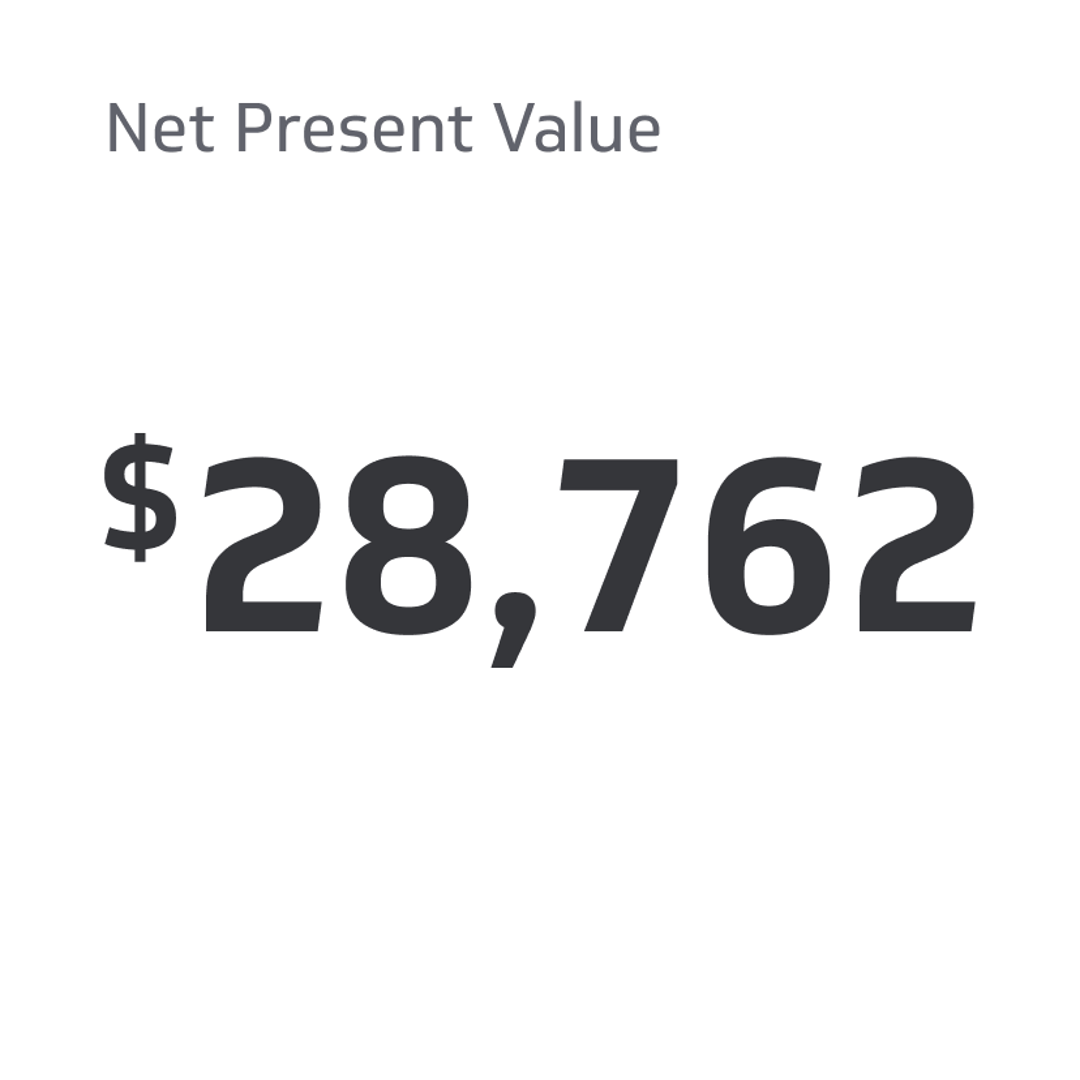
Your business's net present value (NPV) measures its future cash flows compared to the initial investment. By analyzing an NP calculation, you can determine whether a proposed project will likely produce positive returns.

Most businesses use the NPV calculation to evaluate potential investments, projects, or products. The calculation helps you understand what the future cash flows of a project are worth in today's dollars. It also lets you compare projects with different time horizons and cash flow projections.

Whether considering a new business venture or evaluating an existing project, the NPV calculation can help determine if it's worth pursuing.

Keep reading to learn more about the NPV calculation, what it means, and the steps you can take to measure it.

What is Net Present Value (NPV)?

NPV calculation compares a project's expected cash flows against the initial investment required to get it off the ground. It considers the concept of the time value of money or the idea that a dollar today is worth more than a dollar in the future.

In other words, an investment that returns $110 a year from now is not worth the same as if it returns $100 today. The $110 a year from now would be worth less today than the $100 returned immediately.

Accounting for the time value of money helps you determine a project's expected returns in present-day dollars.

What Is a Good Net Present Value?

A good NPV result produces a positive return. Theoretically, any project with a positive NPV should be accepted as it is expected to generate returns above the initial investment.

However, your business may have other factors to consider before accepting a project. For example, compare the NPV of two projects and choose the one with the higher return.

You should also set a higher margin of safety when evaluating projects with a longer timeline or uncertain cash flow projections. For instance, you may only accept projects with an NPV of at least 10% instead of one that generates a 5% return.

A higher margin of safety reduces the risk associated with a project, allowing you to make more informed decisions. You may also consider other factors, such as the project's risk profile and conditions.

How to Measure Net Present Value

To calculate NPV, you must know the initial investment and the expected cash flows.

Once you have this information, you can use the following formula:

NPV = Initial Investment + (Annual Cash Flow / (1 + Discount Rate))

The discount rate should reflect the amount you would invest in a risk-free investment, such as a government bond.

For example, if you were calculating the NPV of a project with an initial investment of $3,000 and an expected cash flow of $1,500 per year for five years with a discount rate of 4%, the calculation would look like this:

NPV = $3,000 + ($1,500 / (1 + 0.04)) = $4,442

In this example, the NPV is $4,442, which means the project is expected to generate a return of $1,442 more than the initial investment of $3,000.

If you're calculating the NPV over a longer time horizon, you must also factor in inflation. This can be done by multiplying the expected cash flow by a certain percentage each year.

For example, if you expect the cash flow to increase by 2% each year due to inflation, you would use the following formula:

NPV = Initial Investment + (Annual Cash Flow / (1 + Discount Rate)) * (1+Inflation Rate)^Years

Using the same example as before, the calculation would look like this:

NPV = $3,000 + ($1,500 / (1 + 0.04)) * (1+0.02)^5 = $4,592

In this example, the NPV is now $4,592, an increase of $150 from the previous example.

By calculating the NPV of a project or investment, you can determine whether it's likely to yield positive returns. If the NPV is positive, you can expect to make a return on your investment.

If the NPV is negative, you can expect to lose money on your investment.

Knowing how to calculate and interpret the NPV of a project can help you make better business decisions and ensure that you're investing in projects that are likely to yield positive returns.

Steps to Measure NPV Using a Spreadsheet

For those who want to measure NPV using a spreadsheet, you can use the following steps:

1. Input the initial investment amount and discount rate into cells A1 and A2, respectively.

2. Enter the expected cash flows for each period in cells A3 to A7.

3. In cell A8, enter the formula “=NPV(A1,A2:A7)”. This will calculate the NPV of the project.

4. The result in cell A8 will give you the NPV.

Remember, when calculating NPV, it's essential to factor in inflation, as this will affect the calculation.

Using the same formula, you can calculate a project's internal rate of Return (IRR) for a project. Simply substitute the NPV function with the IRR function: "=IRR(A1, A2:A7)". It will give you the IRR of the project.

By calculating the NPV and IRR of a project, you can make better decisions about whether to invest in it. If both values are positive, the project will generate a positive return on investment.

Examples of Net Present Value

To illustrate the concept of NPV, consider the following examples.

Example 1: You invest $2,000 in a project and expect to receive $3,000 in cash flows over the next five years.

Using the formula above, you would calculate the NPV as follows:

NPV = $2,000 + ($3,000 / 0.04) = $8,250

In this example, the NPV is $8,250, meaning the project is expected to generate a positive return of $6,250.

Example 2: You invest $2,000 in a project and expect to receive $3,000 in cash flows over the next five years with an inflation rate of 2%.

Using the formula above, you would calculate the NPV as follows:

NPV = $2,000 + ($3,000 0.04) * (1+0.02)^5 = $8,805

In this example, the NPV is $8,805, which means the project is expected to generate a positive return of $6,805.

If the expected cash flows in either example had been negative, the NPV would have been negative, indicating that the project would likely yield a negative return investment.

You can also use NPV to compare two different investments. For example, if you had two projects with the same expected cash flows but various initial investments and discount rates, the higher NPV would be the more profitable option.

Challenges of Using NPV to Make Investment Decisions

Although NPV is a helpful tool for measuring the potential return on investment, some challenges should be considered when making decisions.

First, NPV calculations are based on assumptions about cash flows and discount rates. If these assumptions are accurate or change over time, the NPV calculation will be accurate.

Second, calculating the NPV requires careful consideration of both the expected returns and risks associated with a project. It's essential to consider both factors when calculating the NPV, as an overly optimistic estimate of expected returns may lead to inaccurate NPV calculation.

Finally, NPV is a time-dependent measure and doesn't consider that cash flows may not all be received simultaneously. For instance, if you run a business and expect cash flows to be received evenly over a year, the NPV calculation may need to accurately reflect the project's actual value.

In conclusion, Net Present Value is a valuable tool for measuring the potential return on investment of a project or investment. With careful consideration of assumptions and risks, it can be a helpful way to make investment decisions.

When using NPV in decision-making, it's important to remember that it is based on assumptions and can be affected by changes in circumstances.

Complementary to NPV Calculations

Making sound investment decisions also requires understanding the risks associated with a project or investment. Risk assessment tools, such as Monte Carlo simulations sensitivity analysis, can be used with NPV calculations to provide a more comprehensive view of the potential returns and risks.

The simulations can offer insights into how different variables, such as cost and timing of cash flows, can affect the potential returns. Additionally, sensitivity analysis helps to identify which parameters are most sensitive to changes in expected cash flows and, therefore, should be included when making investment decisions.

These additional tools offer valuable insight into the potential returns of project investment and can help inform decisions. For instance, if a Monte Carlo simulation indicates a high probability of a negative return, it may be prudent to avoid investing in the project.

Other essential risk assessment tools, such as Value at Risk (VaR) and Expected Shortfall (ES), can provide additional information about the potential loss. VaR estimates the maximum loss an investment could suffer in a given period, while ES measures the expected amount of losses beyond VaR. These tools can provide valuable insight into the potential risks associated with investment and help inform decisions.

Regardless of the decision-making tool used, it's important to remember that investments are inherently risky and should be carefully evaluated. By understanding the risks associated with a project or investment and utilizing tools such as NPV and risk assessment, investors can make informed decisions that will help maximize returns.

Whether you are an individual investor or a business owner, understanding and utilizing the concepts of NPV and risk assessment can help ensure that your investments are sound.

Net Present Value Vs. Internal Rate of Return

Net Present Value (NPV) and Internal Rate of Return (IRR) are two commonly used metrics for measuring the potential returns of an investment. While the two calculations share some similarities, they have essential differences.

NPV is a measure of the discounted value of cash flows over some time. It takes into account the present value of future cash flows, considering factors such as inflation and tax rates.

On the other hand, IRR is a measure of returns that focuses on the rate at which invested capital grows over time and does not consider the present value of future cash flows.

The main difference between NPV and IRR is that NPV discounts future cash flows while IRR does not. It means that NPV is more accurate when used to measure investments with uneven cash flows, such as those associated with project investments.

In addition, NPV is more accurate when used to compare projects with different lifetimes or risk profiles. It makes comparing investments with different durations easier, as the present value factor is applied uniformly across all cash flows.

Overall, NPV is the preferred method of analysis for measuring the potential returns of an investment. It considers essential factors such as inflation, tax rates, and the present value of future cash flows. It also allows for easier comparison between investments with different durations or risk profiles.

Net Present Value Vs. Payback Period

The payback period is another commonly used metric for evaluating the potential returns of an investment. Unlike NPV, which considers the present value of future cash flows, the payback period measures the time it takes for an investment to recoup its initial cost.

The payback period is calculated by dividing the initial cost of an investment by the annual cash flow generated from that investment. The result is expressed in years; the shorter the payback period, the better.

However, there are several drawbacks to using the payback period to measure potential returns.

First, it needs to consider the present value of future cash flows, making it an incomplete measure of returns. Second, it does not consider the risk associated with the investment, making comparing investments with different risk profiles challenging.

Finally, it does not consider the time value of money, meaning it does not account for inflation.

NPV is a more accurate and comprehensive measure of returns than the payback period. If you are looking for a precise estimate of returns, then NPV is the preferred financial metric.

Conclusion

Whether you're an individual investor or a business owner, understanding and utilizing the concepts of Net Present Value (NPV) and risk assessment can help ensure that your investments are sound. 

NPV is a more accurate and comprehensive measure of returns than the payback period, considering important factors such as inflation, tax rates, and the present value of future cash flows. It also allows for easier comparison between investments with different durations or risk profiles. 

By understanding and leveraging these concepts, you can make informed decisions about where to allocate your resources for maximum returns.

Learn more about NPV and risk assessment today to get the most out of your investments.

FAQs

Calculating the net present value of an investment requires a few simple steps. Most people ask the following questions:

When calculating NPV, what are the factors that need to be taken into account?

When calculating NPV, you need to consider the present value of future cash flows, inflation, tax rates, and risk associated with the investment. You also need to consider the time value of money, which considers inflation.

What are the benefits of using NPV as a measure of returns?

The benefits of using NPV as a measure of returns include accuracy and comprehensiveness. NPV considers essential factors such as inflation, tax rates, and the present value of future cash flows. It also allows for easier comparison between investments with different durations or risk profiles.

When should I use the payback period to evaluate an investment?

The payback period is best suited for investments with shorter durations. It does not consider the present value of future cash flows or inflation, making it an incomplete measure of returns. For a more accurate and comprehensive estimate of returns, you should use NPV.